Remerciez-le!

Remerciez @Admin pour avoir partagé cet document gratuitement, de la manière la plus simple, en partageant sur les réseaux sociaux.

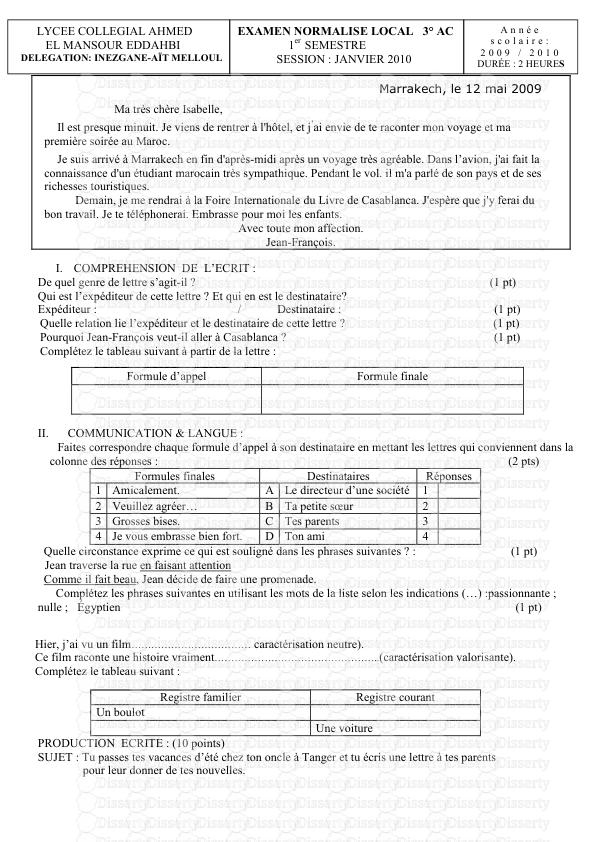
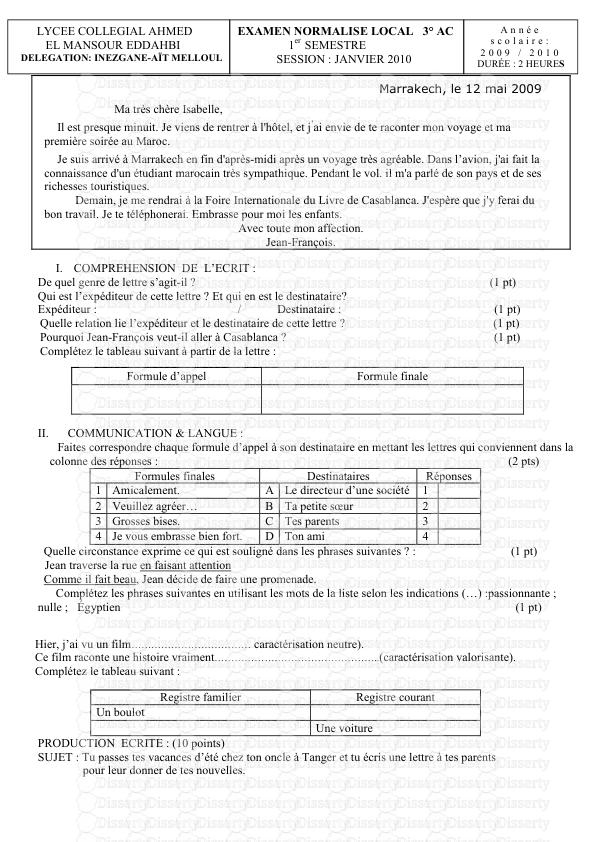
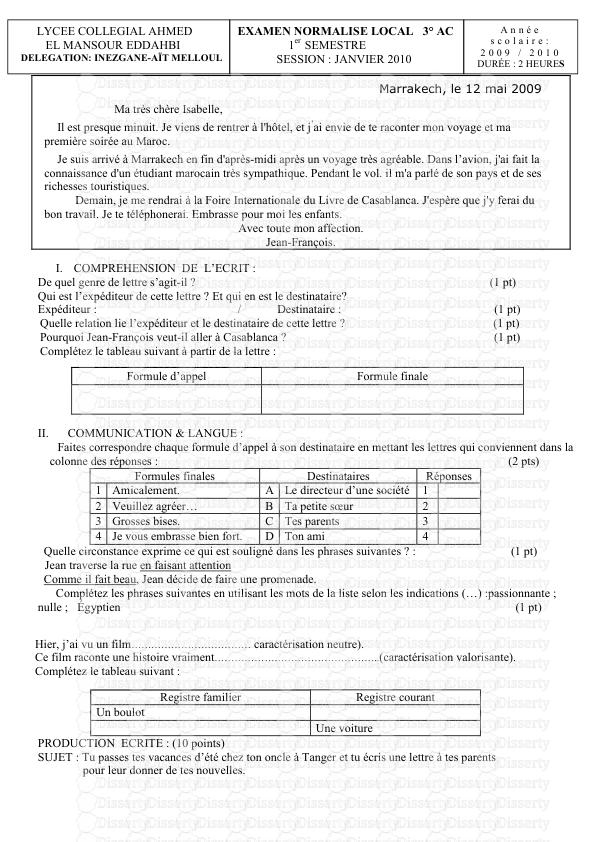
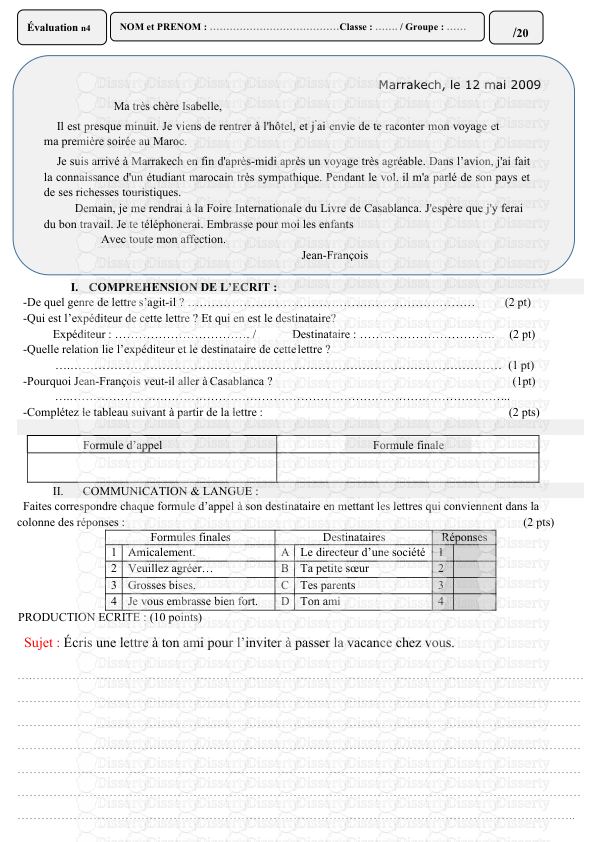
La French-C4D présente : (French-C4D team presents) WIP - Tutorial « Formules »

La French-C4D présente : (French-C4D team presents) WIP - Tutorial « Formules » avec par ordre alphabétique (with alphabetically) Base80 Joscar Amell Karfax deck luistappa MrBoO rmd SoKoa Cette petite histoire a commencé par un défi technique qui a été transformé en aventure dans le domaine des formules mathématiques. Nous espérons que vous apprécierez votre voyage ! :) This small story started with a technical challenge which was transformed into an adventure in the field of the mathematical formulas. We hope you'll enjoy your trip ! :) 2 Challenge Résultat/Result 3 Comment ?/How ? Preview Formule Technique Après avoir obtenu la base, l’épaisseur est obtenue en éditant la sphère et en utilisant le plug-in Make Thicker. Final sphere is obtained by editing the formula, and with the use of Make Thicker plug-in. 4 Détails & explorations 5 6 7 8 9 10 11 12 13 14 15 16 sin((u+t)*2.0*pi)*cos(v+t)*2*pi) 17 X : 260*sin(6*t * 8 ) Y : (300*cos(1.5 *t))*sin(t) Z : 200*sin(1.01*t) Tmin : -85 Tmax : 30 Echantillon : 120 x(t)=100*Cos(t)*Sin(t) y(t)=50*Cos(t*2) z(t)=80*Sin(t*pi)*Cos(t*pi) t-min=-11 t-max=11 echantillons=200 x(t)=30*t*Cos(t) y(t)=400*Cos(t) z(t)=600*Sin(t)*Sin(t)*Cos(t*2) t-min=-20.4 t-max=20.4 echantillons=200 18 Preview Formule Technique 19 20 21 Preview Technique Formule 22 Technique Preview Formule 23 X(t)=(150*sin(20*t))*cos(t*2*pi) Y(t)=0 Z(t)=(150*sin(20*t))*sin(t*2*pi) X(t)=(200*sqrt(abs(cos(2*t))))*cos(t*2*pi) Y(t)=0 Z(t)=(200*sqrt(abs(cos(2*t))))*sin(t*2*pi) X(t)=(100*(1+cos(t)))*cos(t*2*pi) Y(t)=0 Z(t)=(150*sin(20*t))*sin(t*2*pi) X(t)=(100*(1+cos(t)))*cos(t*2*pi) Y(t)=0 Z(t)=((100*(1+cos(t)))*sin(t*2*pi) 24 Preview Formule Technique 25 26 Preview Formule Technique Preview Formule Technique (DiTools Parametric) 27 (DiTools Parametric) 28 http://www.3d-meier.de/ http://www.cs.technion.ac.il/~cs234326/projects/EscherStripes/EscherStripes.html http://school.ort.spb.ru/library/thinkquest98/story1/page2/page2.htm http://mathworld.wolfram.com/SphericalSpiral.html http://www.owlnet.rice.edu/~fjones/loxo.html http://gianpf.free.fr/ http://www.mathcurve.com/courbes3d/solenoidtoric/solenoidtoric.shtml http://www.c4d-treff.de/tutorials/ http://gianf.free.fr/mathematica/indexmathematica.html http://www.frenchcinema4d.com/phpBB2/viewtopic.php?t=4546&highlight=moebius http://www.math.toronto.edu/~drorbn/KAtlas/Knots/index.html http://www.math.toronto.edu/~drorbn/KAtlas/TorusKnots/index.html http://www.pims.math.ca/knotplot/download.html http://perso.wanadoo.fr/math-art http://www.remotion.de.vu/ Liens/Links uploads/Voyage/ c4d-utilisation-des-formules.pdf